Basic Electronics on the Go - Passive Band Pass RC Filter

From http://www.electronicshub.org/passive-band-pass-rc-filter/

Introduction

We can say that a Band pass filter is a combination of both low pass filter and high pass filter. The name of the filter itself indicates that it allows only a certain band of frequencies and blocks all the remaining frequencies. In audio applications, sometimes it is necessary to pass only a certain range of frequencies - this frequency range does not start at 0Hz or end at very high frequency but these frequencies are within a certain range, either wide or narrow. These bands of frequencies are commonly termed as Bandwidth.

Passive Band Pass filter

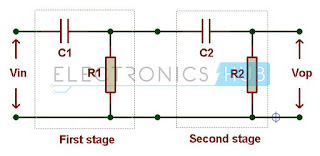
Band pass filter is obtained by cascading passive low pass and passive high pass filters. This arrangement will provide a selective filter which passes only certain frequencies. This new RC filter circuit can pass either a narrow range of frequencies or wide range of frequencies. This range of frequencies that is either narrow or wide range will depend upon the way the passive low pass and high pass filter cascade. The upper and lower cut-off frequencies depend on filter design. This band pass filter is simply  like a frequency selective filter.

The above figure shows the Band pass filter circuit. The input given is a sinusoidal signal. The properties of low pass and high pass combinations give us Band pass filter. By arranging one set of RC elements in series and another set of RC elements in parallel the circuit behaves like a band pass filter. This gives us a second order filter because the circuit has two reactive components. One capacitor belongs to the low pass filter and another capacitor belongs to the high pass filter. Without any variations in the input signal this band pass filter will pass a certain range of frequencies. This filter does not produce any extra noise in the signal. The cut-off frequency of the circuit can be calculated as follows

fC = 1/(2πRC)​

By adjusting the cut-off frequencies of the high pass and low pass filters we can obtain the appropriate width of the pass band for the band pass filter.

Since this filter passes a band of frequencies this filter contains two cut off frequencies, lower cut-off frequency ‘ fL‘ and higher cut-off frequency ‘fH’. Thus the range of the frequencies which are passed through the filter is called as Band Width of the filter. In general the Band Width of the circuit can be calculated by the frequencies ‘fH and fL‘.

BW = fH – fL​

Where , ‘fH‘ is the cut-off frequency of the high pass filter and  ‘ fL‘ is the cut-off frequency of the low pass filter. ‘BW’ is the bandwidth of the filter. The band pass filter will pass the frequencies higher than the cut off frequency of the high pass filter and lower than the cut off frequency of the low pass filter. This shows that the cut off frequency of the low pass filter must be higher than the cut off frequency of the high pass filter.

Band pass filter using R, L and C components

Band Pass Filter circuit design by using inductor, capacitor and resistor is as shown below

The centre frequency of the band pass filter which is also termed as ‘resonant peak’ can be formulated by using the equation.

fc = 1/2π√(LC)​

Where L = inductance of an inductor whose units are in Henry (H).

C = capacitance of a capacitor whose units are in Farad (F).

We can also design a band pass filter with inductors, but we know that due to high reactance of the capacitors, the band pass filter design with RC elements is more advantageous than RL circuits.

The pole frequency is approximately equal to the frequency of the maximum gain.

The frequency response curve of the band pass filter is as shown below: The ideal characteristics and the practical characteristics of the band pass filters are different because of the input reactance of the circuit.

The gain of the input signal can be calculated by taking 20 log (Vout / Vin). The range can be quite large, depending on the  inherent characteristics of the circuit. The signal is attenuated at low frequencies with the output increasing at a slope of +20 dB per decade or 6 dB per octave until the frequency reaches to lower cut off frequency ‘fL’. At this frequency the gain of the signal is at the value 1/√2 = 70.7%.

At maximum gain, the  gain is constant until it reaches the higher cut off frequency ‘f_H’.After the higher cut-off frequency, the output decreases at a slope of -20dB/decade or -6dB/octave.

Previously we have seen that the phase shift of  the first order filter is 90°. We know that the band pass filter is a second order filter so the phase shift is twice of the first order filter that is 180°. The phase angle will vary with the increase of the frequency. At centre frequency the output and input signals are in-phase with each other. Below the resonant frequency the output signal leads the input signal and above the resonantfrequency the output signal lags the input signal. The amplitude of the input signal is always greater than the output signal. In order to increase the gain of the circuit the resistance R1 value must be higher than the resistance R2.

Band Pass Filter Centre Frequency

The “Center frequency” or “Resonant frequency” at which the output gain is maximum can be obtained by calculating the Geometric mean of lower and upper cut-off frequencies.

fr2 = fH x fL

fr = √(fH x fL)

Where fr is the resonant frequency or centre frequency

fH  – is the upper -3 dB cut-off frequency

fL – is the lower -3 dB cut-off frequency

Passive Band Pass Filter Summary

The band pass filter is obtained by cascading a low pass and high pass filter. It is a second order filter since it contains two reactive elements. The order of the filter depends on the number of cascading circuits using in the circuit.

The gain of the output signal is always less than the input signal.At the centre frequency the output signal is in-phase, but below centre frequency the output signal leads the phase with shift of +90° and above centre frequency the output signal will lag in phase with the phase shift of -90°.

The practical characteristics of the band pass filter are a bit different with respect to the ideal characteristics. This variation is mainly due to cascading of high pass filter with low pass filter. The output gain is always less than unity. When we provide electrical isolation between the high pass and low pass filters we can attain better performance of the filter.

The band pass filter will optimize the sensitivity of the receiver. The high pass filter is first added to the design later low pass filter is added. Even though we add a low pass filter first and then the high pass filter, it will never make changes in the output signal. The quality factor of the filter will depend upon the resistor value R1. If R1 is low the quality factor is low and if the R1 value is high then, the quality factor is high.

Applications of Band Pass Filter

1. These are used in wireless communication medium at transmitter and receiver circuits. In transmitter section this filter will pass the only required signals and reduces the interfering of signals with other stations. In the receiver section, it will prevent unwanted signal penetration in to the channels.

2. These are used to optimize the signal to noise ratio of the receiver.

3. These are used in optical communication area like LIDARS.

4. They are used in some of the techniques of colour filtering.

5. These are also used in medical field instruments like EEG.

6. In telephonic applications, at DSL to split phone and broad band signals.

Basic Electronics on the Go - Passive High Pass RC Filters

From http://www.electronicshub.org/passive-high-pass-rc-filters/
From http://www.electronics-tutorials.ws/filter/filter_3.html

Electrical filter is a circuit, designed to reject all unwanted frequency components of an electrical signal and allows only desired frequencies. In other words a filter is a circuit which allows only a certain band of frequencies.

In many applications, capacitive filters are used more than the inductive filters because the inductors will produce some stray magnetic fields and dissipates some amount of power. Not only do they  have these drawbacks but also due to the usage of inductors in the circuit, the filters becomes bulky. In the previous tutorials we have studied basics of filters and passive low pass filter. Now let us see the operation of passive high pass RC filter.

Passive High Pass Filter

The passive High pass Filter is similar to the Passive low pass filter. When the capacitor and resistor positions are interchanged in the circuit of the low pass filter the behaviour of high pass filter is exhibited by the circuit. The capacitor is connected in series with the resistor. The input voltage is applied across the series network but the output is drawn only across the resistor.

High Pass filter allows the frequencies which are higher than the cut off frequency ‘fc’ and blocks the lower frequency signals. The value of the cut off frequency depends on the component values chosen for the circuit design. These high pass filters have many applications at high frequency ranges of 10 MHz.

The circuit of the high pass filter is shown below

Due to this interchange of components in the circuit, the responses delivered by the capacitor changes and these changes are exactly opposite to the response of the low pass filter. The capacitor at the low frequencies acts like an open circuit and at higher frequencies which means at the frequencies higher than the cut off frequency capacitor acts like a short circuit. The capacitor will block the lower frequencies entering into the capacitor due to the capacitive reactance of the capacitor.

We know that the capacitor itself opposes some amount of current through it in order to bind within the capacitance range of the capacitor. After the cut off frequency, the capacitor allows all the frequencies because of the reduction of the capacitive reactance value. This makes the circuit pass the entire input signal to the output when input signal frequency is greater than the cut-off frequency fc. At lower frequencies the reactance value increases thus when reactance increases, the ability to oppose the current flow through the capacitor increases.The band of frequencies below the cut off frequency is referred as ‘Stop Band’ and the band of frequencies after the cut off frequency is referred as ‘Pass Band’.

In the above circuit there is only one reactive component with resistor this shows the circuit is first order circuit.

Frequency Response of high pass filter

The response curves with respect to frequency and the capacitive reactance are given below:

This response curve shows that the high pass filter is exactly opposite to the low pass filter. In high pass filter till the cut off frequency, all the low frequency signals are blocked by the capacitor resulting in the decrease of output voltage. At the cut off frequency point, the value of the resistor ‘R’ and the reactance of the capacitor ‘X_c’are equal thus the output voltage increases at a rate of -20 dB/decade and the output signal levels are -3 dB of the input signal levels.

At very high frequencies the capacitive reactance becomes zero then the output voltage is same as that of the input voltage that is Vout = Vin. At low frequencies the capacitive reactance is infinity and thus the output voltage is zero because the reactance will block the current entering in to the capacitor.

The output of the high pass filter has the phase shift angle (ø) of +45° at cut-off frequency with respect to input signal.This shows that the output of the high pass filter  leads with reference to the input signal. At high frequencies (f > fC) the phase shift is almost zero meaning both input and output signals are in phase. 

The time taken by the capacitor for charging and discharging of the plates with respect to the input signal results in a phase difference. The resistor in  series with the capacitor will produce the charging and discharging effect. The time constant of a series RC circuit is defined as the time taken by the capacitor to charge up to 63.2% of the final steady state value and is also  defined as the time taken by the capacitor to discharge to 36.8% of steady state value. This is represented by the symbol’τ’. The relationship between the time constant and the cut off frequency is given as follows

Time constant τ=RC= 1⁄2πfc and ω_c= 1/τ = 1/RC.

By this it is clear that the output of the filter depends on the frequencies applied at the input and time constant.

Cut off frequency and the Phase shift

The Cut off frequency or Break point ‘fc’ = 1/ 2πfc

The phase shift (ø) = tan-1 (2πfRc)

High pass RC filter output voltage and gain

Second Order Passive High Pass Filter

By cascading two first order high pass filters, we get a  second order high pass filter. Since it consists of two reactive components that mean two capacitors, it makes the circuit a second order. The performance of this two stage filter is equal to single stage filter but the slope of the filter is obtained at -40 dB/ decade. This is because of the cut off frequency variation. It is more efficient when compared with the single stage high pass filter because it has two storage points. For two stage filter the cut off frequency will depend on the values of two capacitors and two resistors. This is given as follows

fc = 1/ (2π√(R1C1R2C2)) Hz​

Passive High Pass Filter Summary

The high pass filter allows the frequencies greater than the cut off frequency up to infinity. In practical situations infinity does not exists so this infinity value is dependent on the components used in the circuit design.

The band of frequencies allowed by the High pass filter is referred to  as ‘Pass Band’ and this pass band is nothing but the bandwidth of the filter. The band of frequencies attenuated by the filter is known as ‘stop band’.

The cut off frequency is calculated by using the formula ‘fc’ which is shown above. The phase shift of the output signal is leading the input signal with an angle of +45°. The output voltage will depends on the time constant and the input frequency applied to the circuit. The distortions eliminated by the high pass filters are more accurate when compared with the low pass filters because of the high frequencies used in the circuit.

High pass RC Differentiator

For normal sinusoidal wave inputs the performance of the filter is just like the first order high pass filter But when we apply different type of signals rather than the sine waves such as square waves which gives time domain response such as step or impulse as the input signal then the circuit behaves like a Differentiator circuit. A circuit whose derivative of the input is directly proportional to the output of the circuit is called a Differentiator circuit.

Thus when constant input is applied to the circuit the output becomes zero because the derivative of the constant tends to zero.

RC Differential circuit is shown below.

For square wave input signals, the output wave form appears as  short duration pulses. For one complete cycle of input, there are two spike signals with positive and negative pulses. In this process there will be no change in the amplitude of the output signal. If the input signal frequency increases then the width of the pulse at the output increases. The rate of the decay of the spike pulse depends on the time constant.

Applications of the High Pass Filter

• These are used in  audio amplifiers as a coupling capacitor between two audio amplifier stages and in speaker systems to direct the higher frequency signals to the smaller “tweeter” type speakers while blocking the lower bass signals 
• These are used as rumble filters to block the nearby unwanted signals and pass the required signals in the loud speakers.
• These are used in the AC coupling circuits and as  differentiator circuits.
• In the mixing process at each channel strips, these high pass filters are added.
• In  image processing, the high pass filters are used in the process of unsharpening where editing requires a high boosting filter.
• In  image processing, the reduction of noise can be done in either time domain or in the frequency domain. So combining with the low pass filters, these high pass filters are used to enhance, noise suppression and modify the images in the image processing.