Star Delta Transformation
We can now solve simple series, parallel or bridge type resistive networks using Kirchhoff´s Circuit Laws, mesh current analysis or nodal voltage analysis techniques but in a balanced 3-phase circuit we can use different mathematical techniques to simplify the analysis of the circuit and thereby reduce the amount of math’s involved which in itself is a good thing.
Standard 3-phase circuits or networks take on two major forms with names that represent the way in which the resistances are connected, a Star connected network which has the symbol of the letter, Υ (wye) and a Delta connected network which has the symbol of a triangle, Δ (delta).
If a 3-phase, 3-wire supply or even a 3-phase load is connected in one type of configuration, it can be easily transformed or changed it into an equivalent configuration of the other type by using either the Star Delta Transformation or Delta Star Transformation process.
A resistive network consisting of three impedances can be connected together to form a T or “Tee” configuration but the network can also be redrawn to form a Star or Υ type network as shown below.
T-connected and Equivalent Star Network
As we have already seen, we can redraw the T resistor network above to produce an electrically equivalent Star or Υ type network. But we can also convert a Pi or π type resistor network into an electrically equivalent Delta or Δ type network as shown below.
Pi-connected and Equivalent Delta Network.
Having now defined exactly what is a Star and Delta connected network it is possible to transform the Υ into an equivalent Δ circuit and also to convert a Δ into an equivalent Υ circuit using a the transformation process. This process allows us to produce a mathematical relationship between the various resistors giving us a Star Delta Transformation as well as a Delta Star Transformation.
Delta Star Transformation
To convert a delta network to an equivalent star network we need to derive a transformation formula for equating the various resistors to each other between the various terminals. Consider the circuit below.Delta to Star Network.
Compare the resistances between terminals 1 and 2.
Resistance between the terminals 2 and 3.
Resistance between the terminals 1 and 3.
This now gives us three equations and taking equation 3 from equation 2 gives:
Then, re-writing Equation 1 will give us:
Adding together equation 1 and the result above of equation 3 minus equation 2 gives:
From which gives us the final equation for resistor P as:
Similarly, resistor Q and R can be found :-
When converting a delta network into a star network the denominators of all of the transformation formulas are the same: A + B + C, and which is the sum of ALL the delta resistances.
Star Delta Transformation
Star Delta transformation is simply the reverse of above. We have seen that when converting from a delta network to an equivalent star network that the resistor connected to one terminal is the product of the two delta resistances connected to the same terminal, for example resistor P is the product of resistors A and B connected to terminal 1.The value of the resistor on any one side of the delta, Δ network is the sum of all the two-product combinations of resistors in the star network divide by the star resistor located “directly opposite” the delta resistor being found. For example, resistor A is given as:



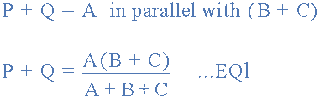












No comments:
Post a Comment